PAST QUESTIONS 2021

Time yourself to improve on your speed. You are to use not more than 60 minutes for this section.

Click on the link below when you are ready.

Kindly contact the administrator of the site on WhatsApp or Phone (0208711375) for the link to the test.

---

Try the questions first, using not more than 15 minutes for each question, and watch the accompanying videos to see how the questions are solved.

---

Question 1

In the Venn diagram, \(M\) and \(N\) are intersecting sets in the universal set \(\mu\).

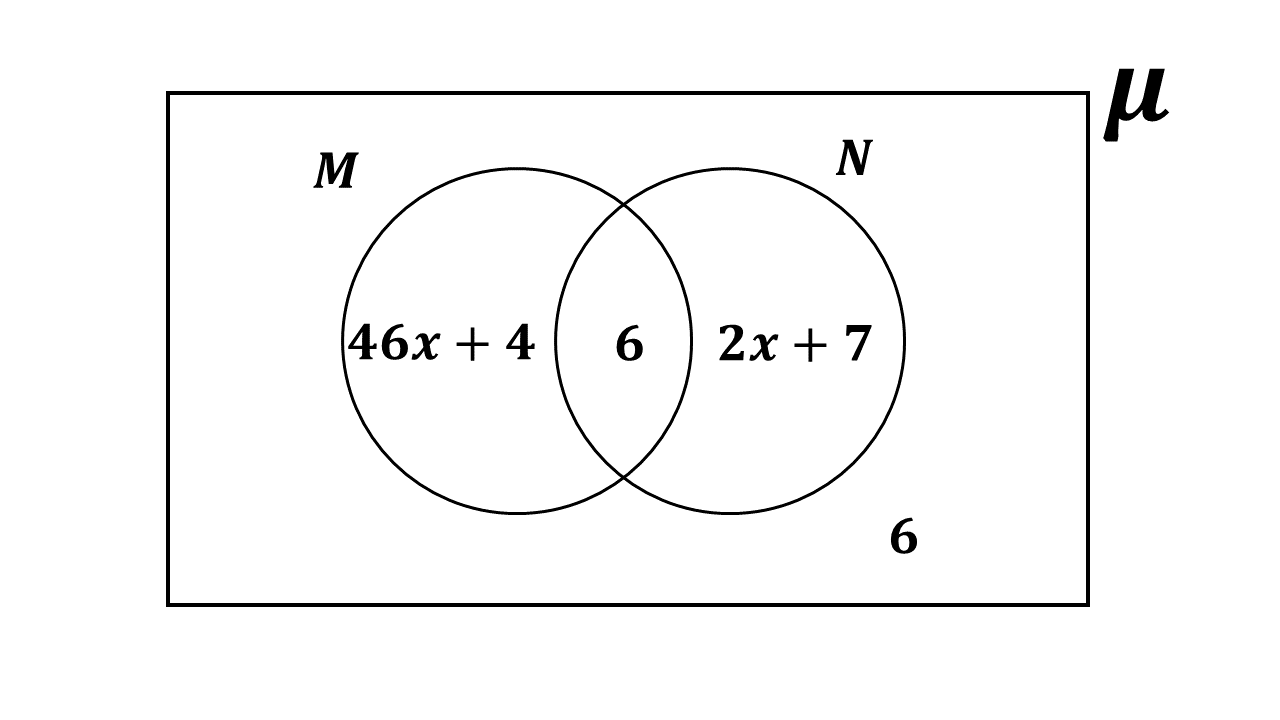
1. Express \(n(M)\) and \(n(N)\) in terms of \(x\).


Show Solution

Solution





2. Given that \(n(M) = n(N)\), find the:

   \((i)\) value of \(x\).

   \((ii)\) \(n(\mathcal{\mu})\).


Show Solution

Solution





3. Simplify: \(2^6 \div (2^2 \times 2^1) \div 2^5\)


Show Solution

Solution

---

Question 2

1. Factorize the expression \(5ay - by + 15a - 3b\).


Show Solution

Solution





2. Solve \(\frac{6}{4p - 1} = \frac{4}{3(p + 4)}\)


Show Solution

Solution





3. Esi and Kofi shared an amount of Gh₵21,000.00 in the ratio of \(2:5\) respectively. How much more did Kofi receive than Esi?


Show Solution

Solution

---

Question 3

1. If \(\mathbf{r} = \begin{pmatrix} -4 \\ -5 \end{pmatrix}\) and \(\mathbf{m} = \begin{pmatrix} -1 \\ -2 \end{pmatrix}\), find \(\mathbf{p}\) given that \(\mathbf{p} = \mathbf{r} - \mathbf{m}\).


Show Solution

Solution





2. The sum of two numbers is 81. If the second number is twice the first, find the second number.


Show Solution

Solution





3. The floor of a rectangular hall is of length 9 m and width 4 m. How may tiles of 20 cm by 30 cm can be used to cover the floor completely?


Show Solution

Solution

---

Question 4

1. Antwiwaa bought 25 mangoes, 7 of which were unripe. What percentage of the mangoes were ripe?


Show Solution

Solution




2. 

The mapping show the relationship between \(x\) and \(y\). Find the

\((i)\) rule of the mapping;

\((ii)\) values of \(m\) and \(n\).


Show Solution

Solution





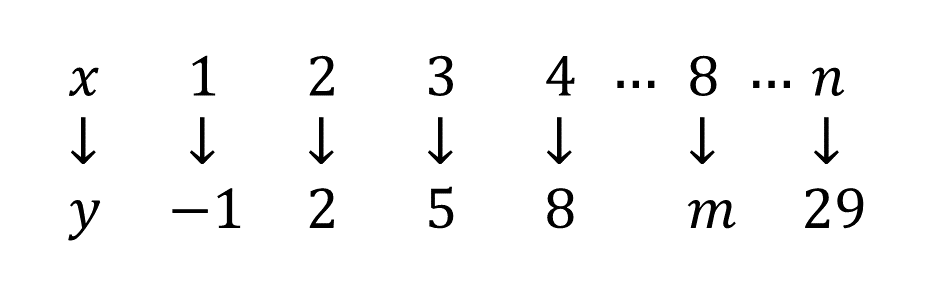
3. A bus left town \(X\) at 6:30 am and arrived at town \(Y\) at 1:00 pm. If the bus travelled at an average speed of 100 km per hour, calculate the distance from town \(X\) to town \(Y\).


Show Solution

Solution

---

Question 5

1. Simplify: \((4x + 2)(x - 2) - 3x^2.\)


Show Solution

Solution





2. The following are the angles formed at the centre of a circle: \(40^\circ, 60^\circ, 100^\circ, 3x^\circ\) and \(5x^\circ\). Find the value of \(x\).


Show Solution

Solution





3. The cost \((C)\) in Ghana Cedis of producing a book of \(x\) pages is given as \(C = 25 + 0.6x\).

   \((i)\) Find the cost of producing a book with 220 pages.

   \((ii)\) How many pages are in a book produced at a cost of Gh₵ 145.00?


Show Solution

Solution

---

Question 6

The table shows the number of marbles students sent to class for Mathematics lesson.

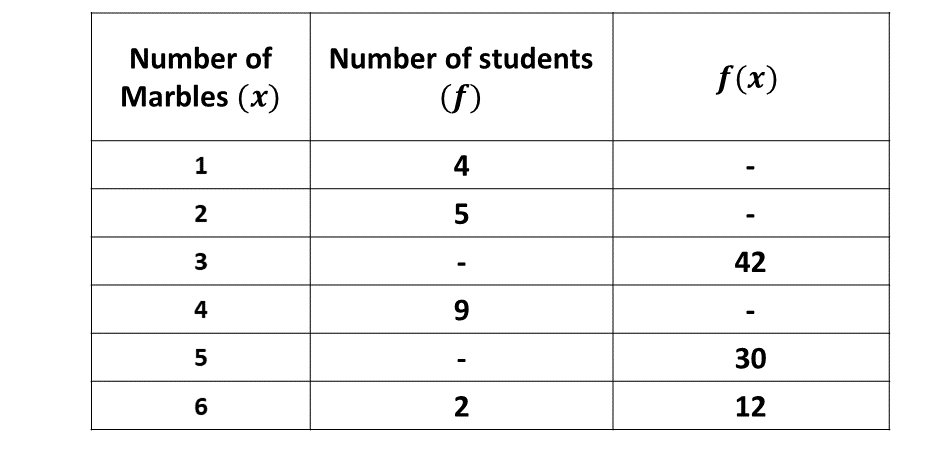
1. Copy and and complete the table.


Show Solution

Solution





2. How many:

   \((i)\) students were in the class?

   \((ii)\) marbles were brought altogether?

   \((iii)\) marbles did most of the students bring?


Show Solution

Solution





3. Calculate correct to the nearest whole number, the mean number of marbles brought for the lesson.


Show Solution

Solution

---