BECE
Year to Practice:
1990
1991
1992
1993
1994
1995
1996
1997
1998
1999
2000
2001
2002A
2002B
2003
2004
2005
2006
2007
2008
2009
2010
2011
2012
2013
2014
2015
2016
2017
2018
2019
2020
2021
2022
2023
2024
PAST QUESTIONS 2005
Time yourself to improve on your speed. You are to use not more than 60 minutes for this section.
Click on the link below when you are ready.
Try the questions first, using not more than 15 minutes for each question, and watch the accompanying videos to see how the questions are solved.
Question 1
-
A boy has enough money to buy 14 pencils at ₵450.00 each. How many erasers costing ₵300.00 each can he buy with the same amount of money?
-
A circle has a circumference of 44 cm. Calculate its radius if \(\pi = \frac{22}{7}\)
-
Simplify \((2\frac{1}{4} - 1\frac{5}{8}) \div 3\frac{7}{16}\)
-
If \(\frac{5(n + 6)}{n} = 1\), find \(n\)
Solution
Solution
Circumference \(= 44\) cm
\(\pi = \frac{22}{7}\)
Circumference of a circle \(= 2\pi r\)
Substituting the given values:
\(\Rightarrow 44 = 2 \times \frac{22}{7} \times r\)
\(\Rightarrow 44 = \frac{44}{7} \times r\)
\(\Rightarrow 44 \times 7 = \frac{44}{7} r \times 7\)
\(\Rightarrow 44 \times 7 = 44 r\)
Dividing both sides by the coefficient
\(\Rightarrow \frac{44 \times 7}{44} = \frac{44 r}{44}\)
\(\Rightarrow r = 7 \)
\(\therefore \) the radius is 7 cm.
Solution
Solution
Question 2
-
Copy and complete the table below for the relation; \(x + y = 180\)
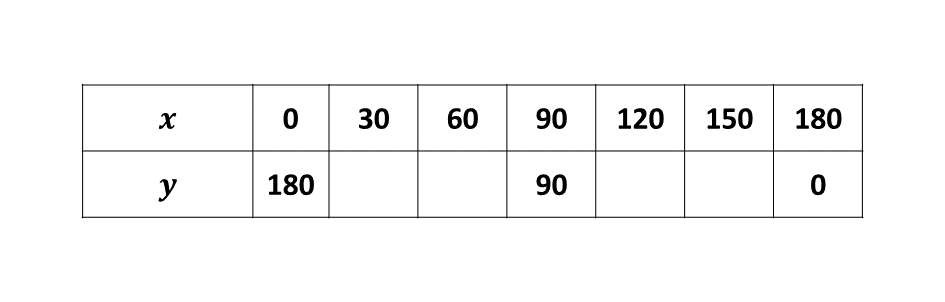
-
\((i)\) Using a scale of 2 cm to 20 units on both axes, draw two perpendicular axes \(Ox\) and \(Oy\).
\((ii)\) Mark both axes from 0 to 180.
\((iii)\) Plot all the seven points. Use a ruler to join the points.
-
Using your graph, find
\((i)\) \(y\) when \(x = 100\);
\((ii)\) \(x\) when \(y = 70\).
Solution
Question 3
-
There are 30 boys in a sporting club. 20 of them play hockey and 15 play volleyball. Each boy plays at least one of the two games.
\((i)\) Illustrate the information on a Venn diagram.
\((ii)\) How many boys play volleyball only?
-
Factorise \(xy + 3x + 6y + 18\)
-
Multiply \((3 + x)\) by \((5 - 2x)\)
Solution
Solution
Solution
Question 4
-
Find
\((i)\) the interest for the 2 years;
\((ii)\) how much she paid in all to the bank after the 2 years.
-
She used the ₵2,000,000.00 to purchase a fridge and sold it at a profit of 45%. Find the selling price of the fridge.
-
If she used the amount raised from the sale of the fridge to pay for the bank loan and interest, find how much money is left.
A woman borrowed ₵2,000,000.00 from a bank at a rate of 15% per annum simple interest for 2 years.
Solution
Question 5
-
Using a pair of compasses and a ruler only, construct
\((i)\) triangle \(ABC\) with \(|AB| = 10\) cm, angle \(ABC = 30^\circ\) and angle \(CAB = 60^\circ\);
\((ii)\) a perpendicular from the point C to meet the line \(AB\) at \(P\).
-
\((i)\) Extend line \(CP\) to the point \(D\) such that \(|BC| = |BD|\).
\((ii)\) Join \(A\) to \(D\) and \(B\) to \(D\)
\((iii)\) Measure \(|AC|\) and \(|AD|\)
-
What type of quadrilateral is \(ADBC\)?
Solution
Question 6
-
Construct a frequency distribution table for the data.
-
What is the modal mark?
-
Calculate the mean mark.
-
How many pupils scored more than 6 marks?
-
What is the probability that a student chosen at random obtained 2 marks?
The following marks were obtained by pupils in a test.
\(\hspace{0.5cm}\)6 \(\hspace{0.5cm}\) 4 \(\hspace{0.5cm}\) 8 \(\hspace{0.5cm}\) 2 \(\hspace{0.5cm}\) 8
\(\hspace{0.5cm}\)6 \(\hspace{0.5cm}\) 8 \(\hspace{0.5cm}\) 8 \(\hspace{0.5cm}\) 8 \(\hspace{0.5cm}\) 10
\(\hspace{0.5cm}\)8 \(\hspace{0.5cm}\) 9 \(\hspace{0.5cm}\) 8 \(\hspace{0.5cm}\) 6 \(\hspace{0.5cm}\) 10
\(\hspace{0.5cm}\)2 \(\hspace{0.5cm}\) 2 \(\hspace{0.5cm}\) 6 \(\hspace{0.5cm}\) 6 \(\hspace{0.5cm}\) 6
Solution
To advertise on our website kindly call on 0208711375 or 0249969740.