BECE
Year to Practice:
1990
1991
1992
1993
1994
1995
1996
1997
1998
1999
2000
2001
2002A
2002B
2003
2004
2005
2006
2007
2008
2009
2010
2011
2012
2013
2014
2015
2016
2017
2018
2019
2020
2021
2022
2023
2024
PAST QUESTIONS 2020
Time yourself to improve on your speed. You are to use not more than 60 minutes for this section.
Click on the link below when you are ready.
Kindly contact the administrator of the site on WhatsApp or Phone (0208711375) for the link to the test.
Try the questions first, using not more than 15 minutes for each question, and watch the accompanying videos to see how the questions are solved.
Question 1
-
If \(M =\) {Prime integers between \(1\) and \(11\)} and \(N =\) {factors of 12}, find:
\((i)\) \(M \cup N\)
\((ii)\) \(M \cap N\)
- Simplify \(45 \div 3 + 2 \times 8 - 12 + 42\)
-
Solution
Solution
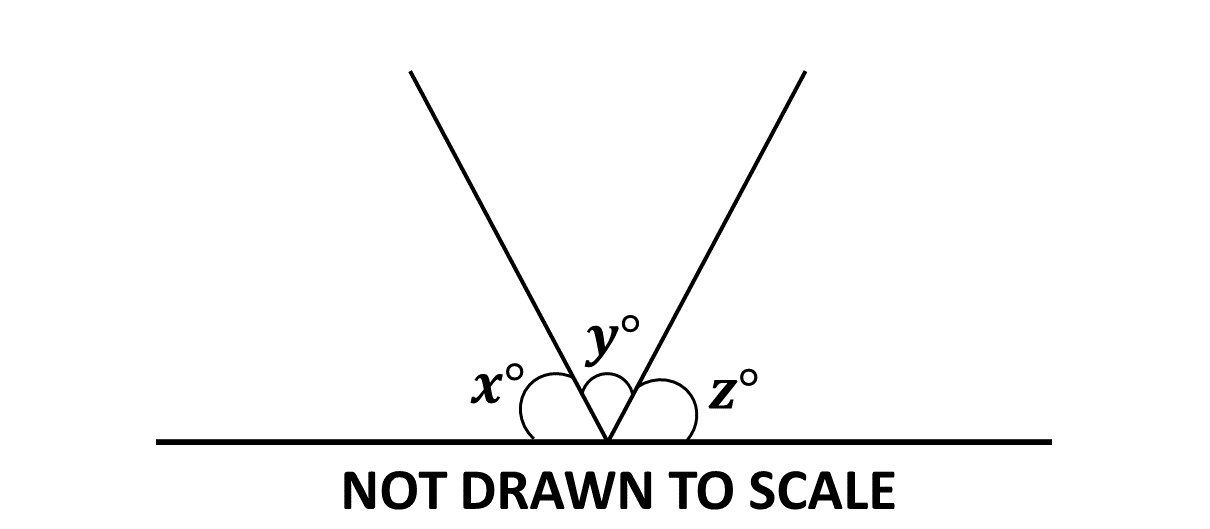
In the diagram, \(x, y\) and \(z\) are angles on a straight line. If \(x^\circ : z^\circ = 2 : 3\), and \(y = 80^\circ\), find \(x\).
Solution
Question 2
-
Simplify: \(5(6 - ab) + 2(-7 + 3ab)\)
-
The equation of a straight line is given by \(3x - 2y - 6 = 0\). Find the:
\((i)\) gradient of the line;
\((ii)\) y-intercept.
-
Adwoa received a commission of 20% on bread she sold. In one week, Adwoa's commission was Gh₵ 540.00.
\((i)\) How much bread did she sell during that week?
\((ii)\) Find her average daily commission.
Solution
Solution
Solution
Question 3
-
Express \((\frac{13}{15} - \frac{7}{10})\) as a percentage.
-
Factorize: \(ay - y - a + 1\).
-
In a fishing community of 9,400 people, the number of women exceeds the number of men by 1,500. Find the ratio of men to women in the community.
Solution
Solution
Solution
Question 4
-
Solve: \(\frac{2x + 3}{3} + 2x = 10\)
-
Multiply 0.03858 by 0.02, leaving the answer in standard form.
-
A cylindrical container of height 28 cm and diameter 18 cm is filled with water. The water is then poured into another container with a rectangular base of length 27 cm and width 11 cm. Calculate the depth of the water in the container. [Take \(π = \frac{22}{7}\)]
Solution
Solution
Solution
Question 5
-
If \(11y = (18)^2 - (15)^2\), find the value of \(y\).
-
Find the perimeter of a circle with radius 35 cm. [Take \(\pi = \frac{22}{7}\)]
-
Given that \(m = \frac{r - s}{2nr}\)
\((i)\) make \(r\) the subject of the relation.
\((ii)\) find the value of \(r\) when \(s = 117, m = 2\) and \(n = -3\).
Solution
Solution
Solution
Question 6
-
Copy and complete the table for the relation \(y = 12x - 9\)
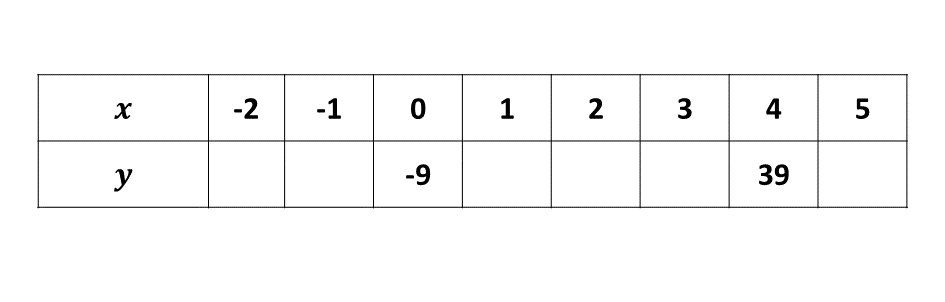
\((i)\) Using a scale of 2 cm to 1 unit on the \(x\) - axis and 2 cm to 10 units on the \(y\) - axis, draw on a graph sheet two perpendicular axes \(Ox\) and \(Oy\).
\((ii)\) Using the table, plot all the points of the relation \(y = 12x - 9\) on the graph.
\((iii)\) Draw a straight line through the points.
\((iv)\) Use the graph to find:
\(\hspace{0.5cm} (\alpha)\) \(y\) when \(x = 2.5\);
\(\hspace{0.5cm} (\beta)\) \(x\) when \(y = 10\);
-
List the integers within the interval \(7 < x \leq 14\)
Solution
Solution
To advertise on our website kindly call on 0208711375 or 0249969740.